
#Mathematica factorial free
For positive arguments, both functions are free of singularities. Here is a quick look at the graphics for the factorial the real axis.Īnd here is a quick view of the bivariate binomial and Pochhammer functions.
#Mathematica factorial series
These symbols are widely used in the coefficients of series expansions for the majority of mathematical functions.Ī quick look at the factorials and binomials

The previous symbols are interconnected and belong to one group that can be called factorials and binomials. For negative integers with, the following definition is used:
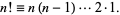
Similarly, for negative integers with, the binomial coefficient cannot be uniquely defined by a limiting procedure based on the previous definition because the two variables, can approach negative integers, with at different speeds. For such integers with, the following definition is used: Remark about values at special points: For and integers with and, the Pochhammer symbol cannot be uniquely defined by a limiting procedure based on the previous definition because the two variables and can approach the integers and with and at different speeds. The first formula is a general definition for the complex arguments, and the second one is for positive integer arguments: The factorial, double factorial, Pochhammer symbol, binomial coefficient, and multinomial coefficient are defined by the following formulas. The classical combinatorial applications of the factorial and binomial functions are the following:57181644131429Item613418010943441Item723121614745161Item Marichev (1994) during the implementation of the function Factorial2 in Mathematica. While the double factorial was introduced long ago, its extension for complex arguments was suggested only several years ago by J. Appell (1880) ascribed the name Pochhammer symbol for the notation of this product because it was widely used in the research of L. Crelle (1831) used a symbol that notates the generalized factorial. von Ettinghausen (1826) later Förstemann (1835) gave the combinatorial interpretation of the binomial coefficients.Ī. Gauss (1812) also widely used binomials in his mathematical research, but the modern binomial symbol was introduced by A. Hindenburg (1779) used not only binomials but introduced multinomials as their generalizations. Euler, who introduced the gamma function as the natural extension of factorial ( ) for noninteger arguments and used notations with parentheses for the binomials (1774, 1781). A special role in the history of the factorial and binomial belongs to L.

Stirling (1730) who found the famous asymptotic formula for the factorial named after him. It was known that the factorial grows very fast. Newton (1676) studied its cases with fractional arguments. Pascal (1653) gave a recursion relation for the binomial, and I. The first mathematical descriptions of binomial coefficients arising from expansions of for appeared in the works of Chia Hsien (1050), al-Karaji (about 1100), Omar al-Khayyami (1080), Bhaskara Acharya (1150 ), al‐Samaw'al (1175), Yang Hui (1261), Tshu shi Kih (1303), Shih–Chieh Chu (1303 ), M. Such combinatorial‐type problems were known and partially solved even in ancient times. The factorials and binomials have a very long history connected with their natural appearance in combinatorial problems. Introduction to the factorials and binomials


 0 kommentar(er)
0 kommentar(er)
